page 25 

Appendix 3: Circular Arrangements of Ley Points

BF 1975; addendum MB 2014

A: The Stonehenge Case

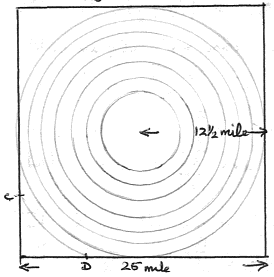
Fig. a.
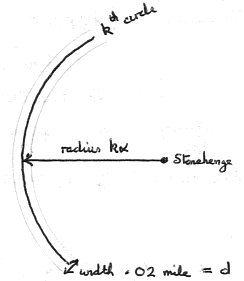
Fig. b.

Here we sprinkle ley points on the map and require to know how many will fall on circles whose radii are multiples of the alpha unit.  In particular, we require to know roughly how many circles will contain two or more points, and also the likelihood that some circle will contain as many as 8 points. 

Stonehenge is assumed at the centre of a 25 mile square area (Fig. a), and complete circles only will be considered – i.e. arcs such as CD will be ignored. 

The alpha unit (α) is 0.18 mile, so the system of complete circles consists of 12.5/0.18=69 circles. 

Each circle has width, like a ley, taken to be 0.02 mile, and the kth circle has radius kα=0.18k miles. 

The ‘corridor area’ for the kth circle (Fig. b) is thus:

2π×0.18k×0.02 square miles.

And the probability p_k that a given ley point will fall on it is:

pk=2π×0.18k×0.02/625=0.000036k

(1)

The probability that r points will fall on the kth circle is:

Pk(r)=nCr pkr (1−pk)n−r

or, since p_k is small for all circles, and n is large:

Pk(r)= (npk)r e−npk . r!

(2)

For this region we take n=1000 (cf. Case 2), and have

npk=0.036k

(3)

By (2) and (3):

Pk(r)= (0.036k)r e−0.036k . r!

(4)

 page 26 

Circles of order at least 2

Let  P_k(0|1) = the probability that the kth circle contains either 0 or 1 point.  By (4)

Pk(0|1)=e−0.036k (1+0.036k).

(5)

Now introduce an indicator variable X_k with

Xk= { 0 if kth ring contains 0 or 1 point 1 if kth ring contains ≥ 2 points

Let

X= ∑ 69 Xk k=1

be the number of circles, out of 69, which contain at least two ley points.  Then if X is the average value of X,

X= ∑ 69 Xk k=1

and

Xk= 0.Pk(0|1)+1.(1−Pk(0|1)=1−Pk(0|1)

whence

X= ∑ 69 (1−Pk(0|1))= 69− ∑ 69 Pk(0|1). k=1 k=1

(6)

By (5)

Pk(0|1)=e−0.036k+0.036ke−0.036k

Letting  x=e^−0.036  we have:

Pk(0|1)=xk+0.036kxk.

Thus (see footnote)

∑ 69 Pk(0|1) = ∑ 69 xk +0.036 ∑ 69 kxk 1 1 1
∑ 69 Pk(0|1) =24.98+19.77=44.75 1	(7)

Substitute (7) in (6):

X=69−44.75=24 to nearest whole number.

That is, we can expect, on average, 24 circles to pass through at least two ley points.  Major Tyler found 23. 

Footnote.  Since x=e^−0.036=0.9646<1, we use

∑ k=n  xk= x(1−xn) k=1 1−x

and

∑ k=n  kxk= x(1−x)[1−(n+1)xn]+x2(1−xn) k=1 (1−x)2

to evaluate (7).  Here, of course, n=69. 

 page 27 

High order circles

Let  P_k(≤r) = probability that the kth circle passes through at most r points. 

Then by (2)

Pk(≤r)=e−0.036k ∑ j=r (0.036k) j  . j=0 j!

(8)

Let  P(all≤r) = the probability that all 69 circles are of order r or less.  Then

P(all≤r)= ∏ k=69 Pk(≤r) . k=1

(9)

[This assumes that the probabilities for the 69 circles are independent, which is not strictly true, of course, though with a large number of ley points, such as we have here (n=1000), the effects of inter-dependence will be relatively small, as in fact computer simulations bear out.]

Let  Q(at least one>r) = the probability that at least one circle out of the 69 contains more than r ley points.  Then

Q(at least one>r)=1−P(all≤r).

(10)

The LHS of (10), of course, is the same as the probability that at least one circle contains at least (r+1) points.  Bearing this in mind we find:

The probability that at least one ring contains at least 7 points	=	0.15
The probability that at least one ring contains at least 8 points	=	0.04
The probability that at least one ring contains at least 9 points	=	0.01

To find a ring of order 8, therefore, is a relatively minor rarity, and it does not even have that distinction if one of the 8 points used was a large point. 

2014: For confirmation by computer simulations, see the update to Appendix 3A

B: The Wooburn Case

Similar to the Stonehenge case except that here the alpha unit restriction is dropped and the circles need not have radii which are multiples of the alpha unit. 

One approach is to set up a system of concentric circles (as in Fig. a above) in which the kth circle has radius 0.02k mile – i.e. the gap between consecutive circles is 0.02 mile or the mean ley-point width.  The centre of any ley point will fall closer to one of the circles than any other, and thus can be considered to fall on that circle.  That is, the centre point will fall within ±0.01 mile of one of the circles somewhere in the system. 

Because the ley points have diameters up to 0.02 mile, this distance is also the minimum distance between circles in order that a ley point becomes associated with only one circle. 

We now have 12½/0.02=625 circles. 

As in the vertex analysis of the leys through Wooburn church, we assume that 400 of the 637 counted ley-point contenders are valid. 

 page 28 

Thus n=400, and using our previous notation np_k=0.00161k.  Following the method in an exactly similar manner we have

X=66 circles,

i.e. without the alpha unit restriction, we can expect to find 66 circles passing through at least 2 ley points. 

Major Tyler produced 25 such circles. 

2014: For an alternative theoretical approach, see the update to Appendix 3B

For the Tyler simulation model

Number of rings =5½/(1/40)=220;  n=150;  np_k=150×2π×0.025×0.025k/121=0.0049k. 

Following our earlier formulae, X=26 in this case.