A ley-hunting lemma

The typescript of these notes is dated 28 Nov 1985. The lemma may be useful in computer simulations of ley-hunting. MB, May 2013

Let K₁,…,K_r be bounded closed convex plane sets, none of which is contained in the intersection of the rest. Suppose there exists a straight line L such that

L∩Ki≠∅ (1≤i≤r).

(1)

Then we may assume that L is an external tangent to two of the K_i.

Proof  Suppose not. Regard L as a movable line. In L, fix an origin O and a direction Ox. Let the words “left” and “right” refer to an observer looking along Ox. Let L∩K_i when non-empty be the interval p_i≤x≤q_i. We show that for any positive integer s there exist a labelling of the K_i and a position of L such that

L is tangent to Ks;	(2)
Ki∩Ki+1=∅ (1≤i≤s−1);	(3)
qi<pi+1 (1≤i≤s−1).	(4)

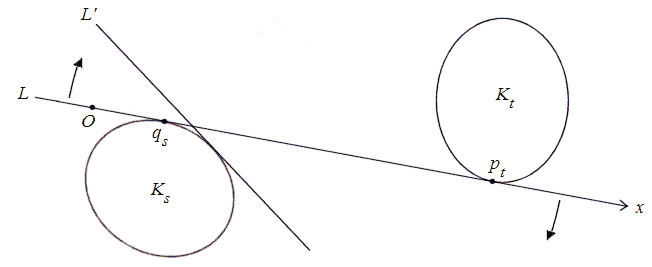
First move L to the left without rotation until (1) fails. In the limiting position L is tangent to some K_t. Label so that t=1; then (2) to (4) hold with s=1.

Suppose (2) to (4) hold for some s. Let K lie to the right (resp. left) of L. Roll L clockwise (resp. anticlockwise) round the boundary of K_s and let θ be the resulting azimuth of Ox.

If (1) holds for all θ then K_s is contained in every other K_i, contrary to hypothesis; hence (1) eventually fails. In the limiting position L is tangent to some K_t with t≠s. By hypothesis [i.e. since the result is assumed false] K_t must lie to the left (resp. right) of L. Hence L can be rolled through a further small angle to a position L′ that separates K_s and K_t with L′∩K_t=∅. Hence K_s∩K_t=0 and q_s<p_t.

As L moves to the limiting position, the p_i and q_i vary continuously with θ, and by (3) q_i≠p_i+1 throughout. Hence at the limiting position (4) holds, so that p_i<p_t for 1≤i≤s. Hence we can label so that t=s+1. This completes the inductive step.

Hence for all s there exist s distinct K_i : contradiction.

Remark  If there are infinitely many K_i the result need not hold; e.g. let K_i be the line segment joining (i,±1/i).